http://people.csail.mit.edu/jaffer/convect
	Convection Articles

• Natural Convection Heat Transfer from an Isothermal Plate
• Skin-Friction and Forced Convection from Rough and Smooth Plates
• Turbulent Mixed Convection from an Isothermal Plate
• Convection Measurement Apparatus and Methodology
• Supplementary Data

Natural Convection Heat Transfer from an Isothermal Plate

Aubrey G. Jaffer

Abstract

Using boundary-layer theory, natural convection heat transfer formulas which are accurate over a wide range of Rayleigh numbers (Ra) were developed in the 1970s and 1980s for vertical and downward-facing plates. A comprehensive formula for upward-facing plates remained unsolved because they do not form conventional boundary-layers.

From the thermodynamic constraints on heat-engine efficiency, the novel approach presented here derives formulas for natural convection heat transfer from isothermal plates. The union of four peer-reviewed data-sets spanning 1<Ra<10¹² has 5.4% root-mean-squared relative error (RMSRE) from the new upward-facing heat transfer formula.

Applied to downward-facing plates, this novel approach outperforms the Schulenberg (1985) formula's 4.6% RMSRE with 3.8% on four peer-reviewed data-sets spanning 10⁶<Ra<10¹².

The introduction of the harmonic mean as the characteristic-length metric for vertical and downward-facing plates extends those rectangular plate formulas to other convex shapes, achieving 3.8% RMSRE on vertical disk convection from Hassani and Hollands (1987) and 3.2% from Kobus and Wedekind (1995).

Full Article

Thermo 2023 3(1): 148-175

arXiv 2201.02612

Natural Convection Heat Transfer from an Isothermal Plate

Supplementary Data

thermo-data.zip

Gray-code (self-similar) ramp-permutation roughness; L/ε≈13.86

Skin-Friction and Forced Convection from Rough and Smooth Plates

Aubrey G. Jaffer

Abstract

Since the 1930s, theories of skin-friction drag from plates with rough surfaces have been based by analogy to turbulent flow in pipes with rough interiors. Failure of this analogy at slow velocities has frustrated attempts to create a comprehensive theory.

Utilizing the concept of a self-similar roughness which disrupts the boundary layer at all scales, this investigation derives formulas for a rough or smooth plate's skin-friction coefficient and forced convection heat transfer given its characteristic length, root-mean-squared (RMS) height-of-roughness, isotropic spatial period, Reynolds number, and the fluid's Prandtl number.

This novel theory was tested with 456 heat transfer and friction measurements in 32 data-sets from one book, six peer-reviewed studies, and the present apparatus. Compared with the present theory, the RMS relative error (RMSRE) values of the 32 data-sets span 0.75% through 8.2%, with only four data-sets exceeding 6%. Prior work formulas have smaller RMSRE on only four of the data-sets.

Full Article

Thermo 2023 3(4): 711-77

arXiv 1810.05743

Skin-Friction and Forced Convection from Rough and Smooth Plates

Supplementary Data

rough-data.zip

Mixed Convection From an Isothermal Rough Plate

Aubrey G. Jaffer and Martin S. Jaffer

Abstract

This investigation derives formulas to predict the mixed convective surface conductance of a flat isotropic surface roughness having a convex perimeter in a Newtonian fluid with a steady forced flow in the plane of that roughness.

Heat transfer measurements of a 30.5 cm square rough plate with forced air velocities between 0.1 m/s and 2.5 m/s were made by the present apparatus in two inclined and all five orthogonal orientations. The present work's formulas are compared with 104 measurements in twelve data-sets. The twelve data-sets have root-mean-square relative error (RMSRE) values between 1.3% and 4% relative to the present theory.

The present work's formulas are also compared with 78 measurements in 28 data-sets on five vertical rough surfaces in horizontal flow from Rowley, Algren, and Blackshaw (1930). The five stucco data-sets have RMSRE values between 2.5% and 6.5%; the other data-sets have RMSRE values between 0.2% and 5%.

Full Article

Thermal Science and Engineering 2025 Vol 8, No 1

arXiv 2401.09469

Mixed Convection From an Isothermal Rough Plate .

Convection Measurement Apparatus and Methodology

Technical details

Wind-tunnel construction

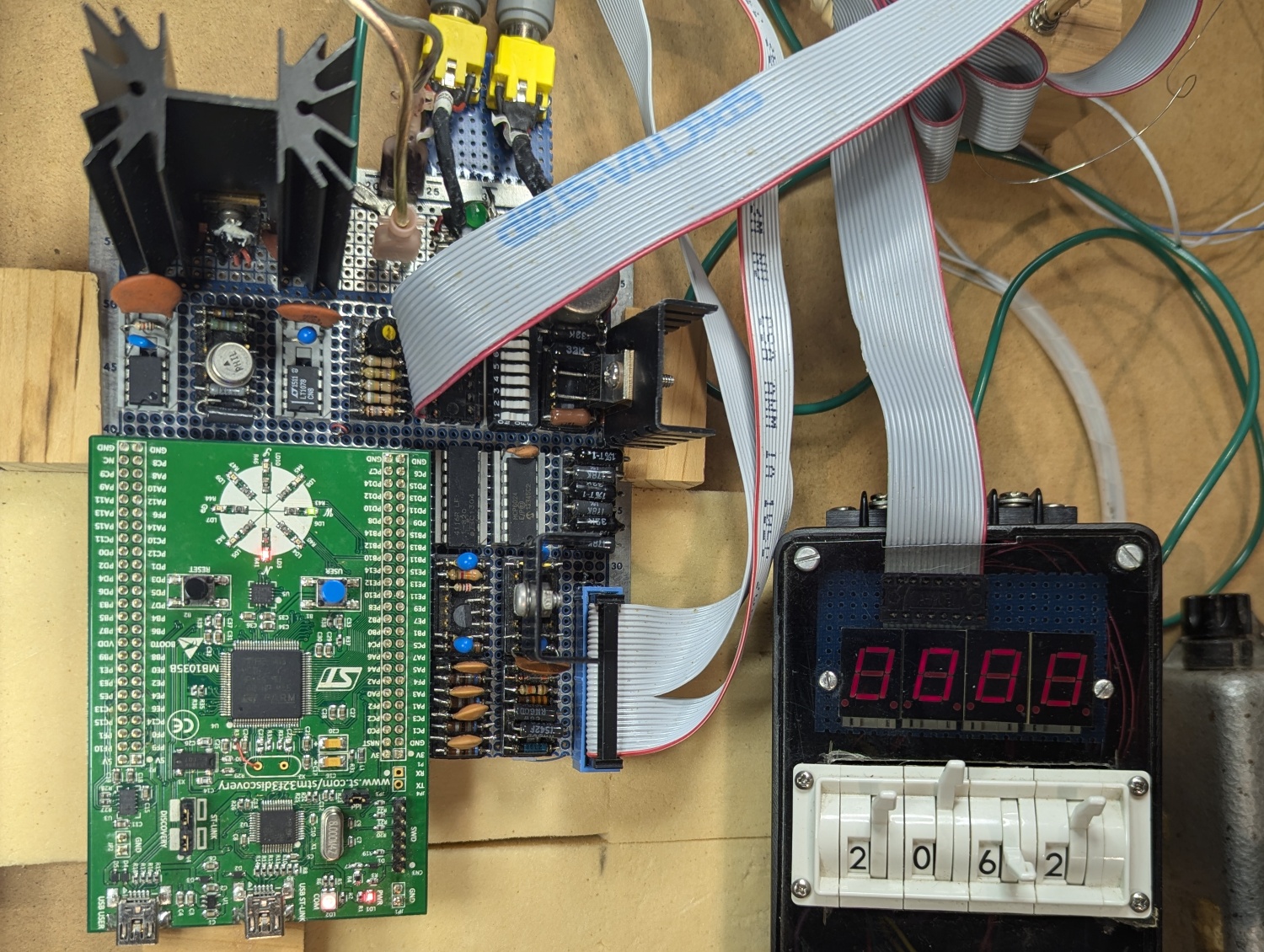
Apparatus electrical schematics (2 page pdf)

STM32F303VCTx configuration (pdf) (included in Firmware.zip)

"convect" source code Firmware.zip creates directory convect; the makefile is convect/Firmware.Makefile.

Apparatus operating instructions

Wind-Tunnel Calibrations

Supplementary Data

In the temperature versus time graphs in the supplementary files, the green, blue, and black traces are the plate, (insulated) back, and ambient temperatures respectively. The upper red trace is a simulation of the plate temperature with the back and average ambient temperatures as inputs. The middle red trace is a simulation of the back temperature with the plate and ambient temperatures as inputs. The lower red line is the ambient temperature averaged over the measurement period.

The diamonds on the plate temperature trace mark the beginning and end of the measurement period, the ending temperature difference with ambient being at most half of the peak temperature difference with ambient.

Underneath each temperature graph is a graph of the air velocity versus time for mixed convection runs and Rayleigh number versus time for natural convection runs.

datafiles.zip has the dimensionless measurements used to generate the summary graphs in each pdf above. The columns are:

1. Re - Reynolds number
2. Ra - Rayleigh number
3. RSS combined measurement uncertainty %
4. θ - surface angle from vertical degrees (−90 is facing up)
5. ψ - flow angle from vertical degrees (0 is up)
6. Pr - Prandtl number
7. Nu - Nusselt number

Characteristic-length L = 0.305 m